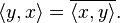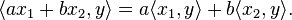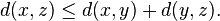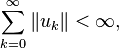Hilbert space

来源：互联网发布：zara淘宝官方旗舰店编辑：程序博客网时间：2024/06/06 19:07

Definition

A Hilbert space H is a real or complex inner product space that is also a complete metric space with respect to the distance function induced by the inner product.^[2] To say that H is a complex inner product space means that H is a complex vector space on which there is an inner product $\langle x,y\rangle$ associating a complex number to each pair of elementsx,y of H that satisfies the following properties:

The inner product of a pair of elements is equal to the complex conjugate of the inner product of the swapped elements:

\langle y,x\rangle = \overline{\langle x, y\rangle}.

The inner product is linear in its first argument.^[3] For all complex numbersa and b,

\langle ax_1+bx_2, y\rangle = a\langle x_1, y\rangle + b\langle x_2, y\rangle.

The inner product of an element with itself is positive definite:

\langle x,x\rangle \ge 0

where the case of equality holds precisely when x = 0.

It follows from properties 1 and 2 that a complex inner product is antilinear in its second argument, meaning that

\langle x, ay_1+by_2\rangle = \bar{a}\langle x, y_1\rangle + \bar{b}\langle x, y_2\rangle.

A real inner product space is defined in the same way, except that H is a real vector space and the inner product takes real values. Such an inner product will be bilinear: that is, linear in each argument.

The norm is the real-valued function

\|x\| = \sqrt{\langle x,x \rangle},

and the distance d between two points x,y in H is defined in terms of the norm by

d(x,y)=\|x-y\| = \sqrt{\langle x-y,x-y \rangle}.

That this function is a distance function means (1) that it is symmetric in x and y, (2) that the distance between x and itself is zero, and otherwise the distance betweenx and y must be positive, and (3) that the triangle inequality holds, meaning that the length of one leg of a triangle xyz cannot exceed the sum of the lengths of the other two legs:

d(x,z) \le d(x,y) + d(y,z).

This last property is ultimately a consequence of the more fundamental Cauchy–Schwarz inequality, which asserts

|\langle x, y\rangle| \le \|x\|\,\|y\|

with equality if and only if x and y are linearly dependent.

Relative to a distance function defined in this way, any inner product space is ametric space, and sometimes is known as a pre-Hilbert space.^[4] Any pre-Hilbert space that is additionally also a complete space is a Hilbert space. Completeness is expressed using a form of theCauchy criterion for sequences in H: a pre-Hilbert space H is complete if everyCauchy sequence converges with respect to this norm to an element in the space. Completeness can be characterized by the following equivalent condition: if a series of vectors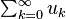 $\textstyle{\sum_{k=0}^\infty u_k}$ converges absolutely in the sense that

\sum_{k=0}^\infty\|u_k\| < \infty,

then the series converges in H, in the sense that the partial sums converge to an element ofH.

As a complete normed space, Hilbert spaces are by definition also Banach spaces. As such they are topological vector spaces, in which topological notions like the openness and closedness of subsets are well-defined. Of special importance is the notion of a closedlinear subspace of a Hilbert space that, with the inner product induced by restriction, is also complete (being a closed set in a complete metric space) and therefore a Hilbert space in its own right.

摘自：https://en.wikipedia.org/wiki/Hilbert_space

0 0